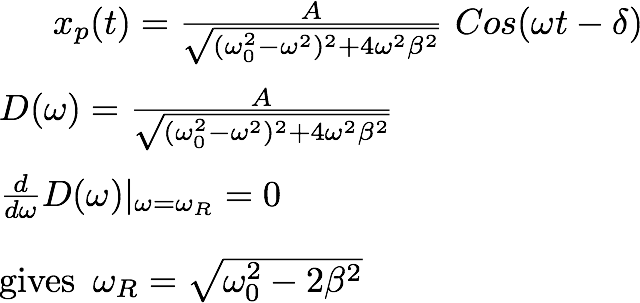DEpartment of physics

In this lecture,we will discuss the effect of driving forces on the damped harmonic oscillator. We talk about the general solution to this problem, and graphicall visualize it.

10.1 Resonance Phenomena

10.1 Resonance Phenomena

In the last lecure,we discussed about the resonance phenomena. Let's work on the math now find out the condition for occuring the resonance. The system is described by a natural frequency and a damping coefficient. We need to find out the frequency of the driving force for this system such that the maximum amplitude of the position coordinate.

that means at this resonance frequency, D is a maximum.

Let's look at the amplitude as a function of frequency, and see the resonance frequency and the maximum amplitude.

The above CDF was created by Thushari Jayasekera

Quality Factor of Resonance

As you can see from the above CDF player, the width of the resonance is charaacerized by the damping coefficient of the oscillator. So we define the Quality Factor of the Driven Oscillator as,

As the damping coefficeint is small, we see sharper resonance. So the Q is larger for sharper resonance.

Let's do few more Problems with Simple Harmonic Oscillator